Aerospaceweb.org | Ask Us - Lift Coefficient & Thin Airfoil Theory

Lift Coefficient & Thin Airfoil Theory

CL = 2 * pi * angle

the angle being expressed in radians. Is this correct?
- question from Chris Warner

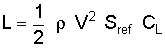
Background information on the lift coefficient and how it is used to calculate the lift force acting on a vehicle is available in previous questions. The key point to understand is that lift is the force that raises an object off the ground and makes flight possible. One of the more common methods of calculating the lift acting on such an object is the equation shown below:

The equation depends on three key quantities:

the operating conditions of velocity (V) and altitude, where altitude defines the atmospheric density (ρ). The velocity and density terms are often combined into a single variable called dynamic pressure (q) to further simplify the equation.
the "size" of the lifting surface defined by the reference area (S_ref).
the "efficiency" or "effectiveness" of the lifting surface defined by the lift coefficient (C_L).

Though at first glance quite simple, the equation is a powerful yet easy-to-use tool in understanding how lift changes under different scenarios. The only problem with the equation is that all of the complex aerodynamics that go into generating lift are hidden away in the somewhat vague lift coefficient variable. What I believe you are asking in your question is if there is a simple and accurate way to determine what the lift coefficient really is.

That leads us to the specific equation you have referenced. What you have stumbled upon is an equation from a simplified approximation called the Thin Airfoil Theory. This theory is handy for learning some basic aerodynamic relationships, but it isn't terribly useful in the real world of engineering because it ignores many of the ugly realities of how the air flowing over a wing really behaves. The particular equation that you mention is an ideal approximation of how the lift coefficient behaves with angle of attack. To be more exact, it is an ideal approximation of the slope of the lift curve, a quantity referred to as C_{L_α} (pronounced "C-L-alpha").

If you remember back to your basic algebra, a straight line can be represented by the equation:

where m is the slope of the line and b where it intercepts the y-axis. The lift coefficient curve behaves in a similar manner and can be represented by an equation of the same form:

where C_{L_α} is the slope of the curve and C_L₀ where it intercepts the y-axis. As you mentioned in your question, α (pronounced "alpha") is the angle of attack in radians.

According to the ideal aerodynamics of the Thin Airfoil Theory, the y-intercept (C_L₀) is 0 and the slope of the lift curve (C_{L_α}) is equal to 2π.

Plugging in those values produces the equation you describe:

The problem with this theory is that it assumes the wing extends to infinity. In other words, the lifting surface has no wingtips. Wingtips introduce a form of drag called induced drag. The stronger the induced drag is, the lower the slope of the lift curve (C_{L_α}) becomes. In addition, Thin Airfoil Theory doesn't account for the fact that the lift coefficient eventually reaches a maximum and then starts decreasing. The angle of attack at which this maximum is reached is called the stall angle. According to Thin Airfoil Theory, the lift coefficient increases at a constant rate--as the angle of attack α goes up, the lift coefficient (C_L) goes up. But in real life, the angle of attack eventually gets so high that the air flow separates from the wing and the wing stalls.

You can better understand the effects of induced drag and stall by studying the following graph.

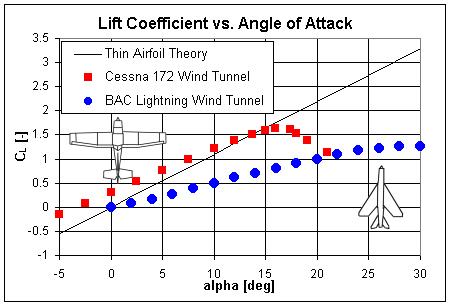
Aircraft lift coefficient data and thin airfoil theory

This graph compares the lift coefficient vs. angle of attack for two actual aircraft, as measured in wind tunnel experiments, compared to the ideal lift coefficient predicted by the Thin Airfoil Theory equation you ask about. You can see that the theoretical equation does approximate the lift coefficient of the Cessna 172 pretty well up to about 15°. At that point, the Cessna's wing stalls and it starts losing lift. The Lightning, on the other hand, doesn't begin to stall until about 27°. However, its lift curve slope is also much lower than Thin Airfoil Theory predicts. This shallow slope results from the fact that the Lightning has what is called a low aspect ratio wing. Aspect ratio is defined as the square of the wingspan (b) divided by the planform area of the wing (S) when viewed from above.

The lower the aspect ratio, the more induced drag a wing will produce, and the lower the lift curve slope becomes.

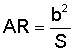
The conclusion to draw from this explanation is that the Thin Airfoil Theory equation can be used to estimate the lift coefficient so long as you understand its limitations. The equation can only be used for aircraft with medium to large aspect ratio wings and only up to the stall angle, which is usually between 10° and 15° for typical aircraft configurations.
- answer by Jeff Scott, 10 August 2003

I have been trying to prove that the equation for lift is accurate. What is the lift coefficient for a Boeing 747? How do you calculate the lift coefficient of an aircraft given values for the other variables? I have tried to work it out but all I have is bad luck.

Aircraft | Design | Ask Us | Shop | Search