Aerospaceweb.org | Ask Us - Airfoils at High Angle of Attack

Airfoils at High Angle of Attack

0° < alpha < 15°

- question from Ramon

Finding tables of airfoil lift and drag characteristics up to angles of attack of 90° is pretty unusual. As you suggest in your question, most tables or graphs of airfoil aerodynamic behavior only go up to the stall angle or a little higher and usually end between 15° and 20°. However, Sandia National Labs conducted some tests in which they collected lift coefficient (c_l) and drag coefficient (c_d) data for seven different airfoils up to angles of attack of 180°. Shown below are the c_l and c_d for the symmetric NACA airfoil section 0015, and the general trends are representative of all the airfoils tested.

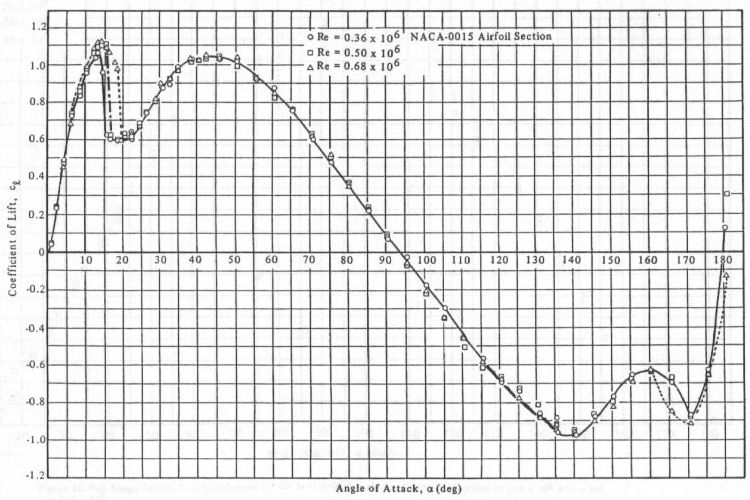
Lift coefficient from 0 to 180 degrees

You'll notice that both coefficients behave much like a flat plate. In fact, beyond the stall angle, all airfoils behave in essentially the same manner as a flat plate. Since the flow becomes separated and the boundary layer is very thick, the actual shape of the airfoil has little or no effect on its aerodynamics in the stalled region. Flat plate aerodynamics predict that the lift coefficient reaches a maximum of 1.2 at 45° before decreasing to 0 at 90°. It is therefore not surprising that the lift curve shown above also indicates a peak of nearly 1.2 at 45° and decreases to 0 close to 90°.

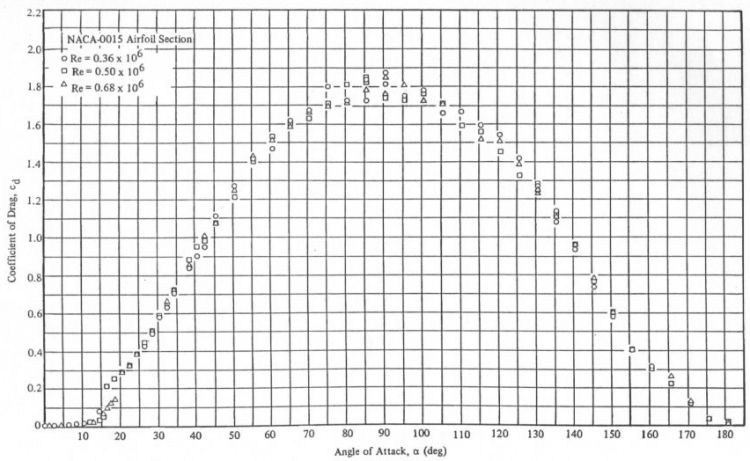
Drag coefficient from 0 to 180 degrees

As for drag, a two-dimensional flat plate at an angle of 90° to the airflow has a c_d of about 2. Since an airfoil section is also a 2D surface, it is not surprising that the c_d vs. alpha also reaches a peak of nearly 2 at 90°.

You can apply these same trends to any airfoil. Most airfoils stall in the region of 15° to 20°. Between 20° and 25°, the c_l begins increasing again to a peak of 1.2 at 45°. The lift then decreases in an approximately linear fashion to 0 at 90°. The trend from 90° to 180° is exactly the same except the c_l is negative. Drag behaves much like a bell curve, with a peak of 2 at 90° while the coefficient is close to 0 at 0° and 180°.

The experimental data shown above is from Sandia National Labs report SAND80-2114 where additional information on this subject can also be found. The report is entitled "Aerodynamic Characteristics of Seven Symmetrical Airfoil Sections Through 180-Degree Angle of Attack For Use In Aerodynamic Analysis of Vertical Axis Wind Turbines" by R.E. Sheldahl and P.C. Klimas. This report can be downloaded at the Sandia Wind Energy Technology site.
- answer by Jeff Scott, 16 November 2003

Aircraft | Design | Ask Us | Shop | Search