Aerospaceweb.org | Ask Us - NACA 0012 Lift Characteristics

NACA 0012 Lift Characteristics

- question from Amos Miles

I'm not sure what Airfoilsim is, but I suspect you may be referring to the Foilsim II applet offered by NASA. Although this applet is a nice tool for learning the basics of aerodynamics, it is somewhat limited in the options it provides to the user. I believe the reason you are seeing such different results is that the applet may not be able to handle such low Reynolds numbers. To obtain a Reynolds number of 179,000 at sea level on an airfoil of unit length, its velocity would only be 19 mph (31 km/h)!

The only experimental data for the NACA 0012 airfoil that I have seen is contained in the classic book Theory of Wing Sections by Abbott and Von Doenhoff. However, this data is at much higher Reynolds numbers of 3 to 9 million. At these conditions, the data suggests the airfoil will stall around 16 degrees. These results appear to agree well with the results provided by Foilsim at comparable conditions. I suspect Foilsim is based on these results and does not use any different lift versus angle of attack data for significantly lower Reynolds numbers.

To investigate your question further, I analyzed the NACA 0012 using two airfoil prediction codes. XFOIL can be downloaded and run as an executable on your computer while Javafoil is a web-based applet. I first used both codes to analyze the airfoil at a Reynolds number of 3 million to compare with the experimental data from Theory of Wing Sections. The results are shown below.

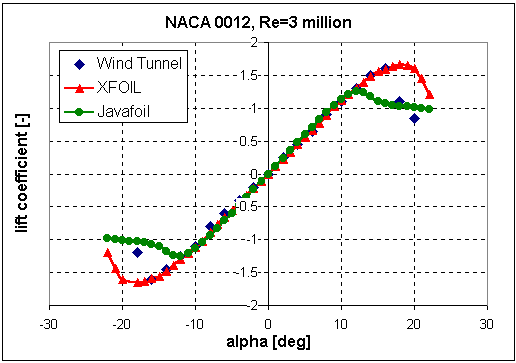
NACA 0012 lift coefficient at a Reynolds number of 3 million

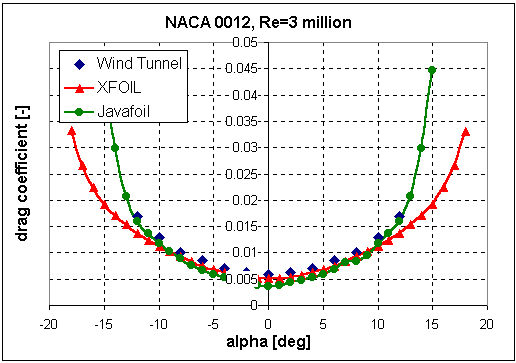
Agreement between XFOIL and the wind tunnel data provided in Theory of Wing Sections is relatively good. The stall angle predicted by XFOIL is about 18°, slightly higher than the experimental value of 16°. The agreement with Javafoil is not quite as good since this code predicts the airfoil will stall at a much lower angle of 12°. While both codes also suggest the stall will be more benign than indicated by the wind tunnel results, the overall agreement between experiment and simulation is reasonably good. A good match is also obtained when comparing the drag coefficient illustrated in the following graph.

NACA 0012 drag coefficient at a Reynolds number of 3 million

These comparisons give us greater confidence in applying the same codes at a Reynolds number of 179,000. The results of the software predictions are compared below to each other and to the original wind tunnel results obtained at a Reynolds number of 3 million. Note how much the stall angle predicted by both codes has decreased compared to the results at the higher Reynolds number.

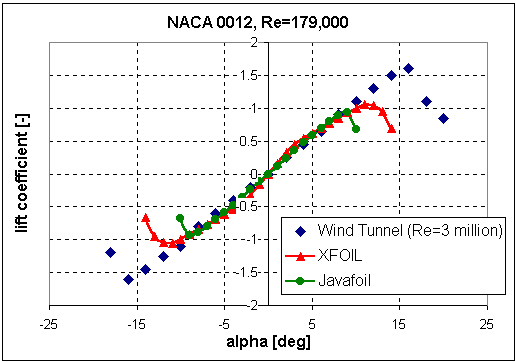
NACA 0012 lift coefficient at a Reynolds number of 179,000

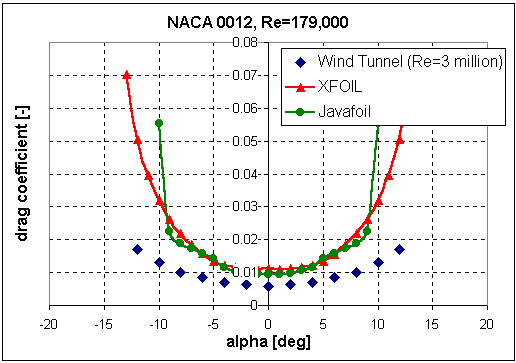
NACA 0012 drag coefficient at a Reynolds number of 179,000

Whereas the airfoil stalled at 16° at a Reynolds number of 3 million, XFOIL now predicts stall will occur at about 11° and Javafoil indicates a stall angle of only 9°. These predictions agree well with the experimental results you describe since they indicate stall occurs at 10°.

However, the lift coefficients predicted by the two codes are closer to 1.0 and considerably larger than the 0.7065 you said the experimental data indicates. A coefficient of 0.7065 sounds much too low for an angle of 10°. We previously discussed a theoretical technique called Thin Airfoil Theory that matches reality quite well for airfoils below the stall angle. This theory tells us that the maximum lift coefficient to be expected at 10° is about 1.1. Both the XFOIL and Javafoil predictions are in agreement with Thin Airfoil Theory, so I find the experimental result questionable. Perhaps the experimental data you have found is for a low aspect ratio wing instead of an infinite airfoil section. Such a wing would generate a reduced lift coefficient slope and create less lift at a given angle compared to an ideal thin airfoil.
- answer by Jeff Scott, 29 January 2006

I was searching for airfoil geometry, and came across equations for NACA 4-Digit airfoils...Can you please explain how these equations are derived or where they come from?

Aircraft | Design | Ask Us | Shop | Search