Aerospaceweb.org | Ask Us - Drag of Cylinders & Cones

Drag of Cylinders & Cones

- question from Rick Briggle

This subject shares some commonality with previous articles in which we discussed the drag coefficient of a sphere and using that of a circular disk to calculate the terminal velocity of a falling penny. In fact, a flat circular disk oriented perpendicular to the airflow is commonly used for calibrating wind tunnel equipment. An example of this application can be found in NACA Technical Note 253, for instance. This shape is so popular because its drag coefficient (C_D) is nearly constant across all operating conditions like Reynolds number. Above a Reynolds number of 1000, the drag coefficient of the circular disk becomes a constant that has been consistently measured as 1.17. This coefficient is based upon a reference area equal to the frontal area of the disk, or π r² where r is the radius of the disk.

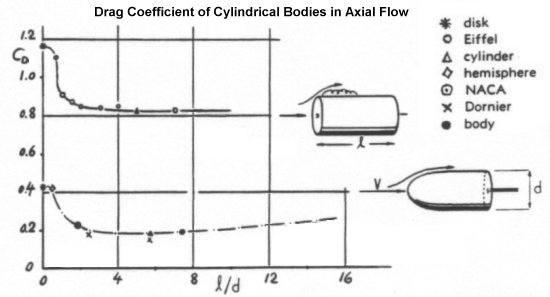
Drag coefficient of blunt nose and rounded nose cylinders versus fineness ratio l/d

Drag coefficient of a circular disk and a sphere versus Reynolds number

It sounds like the shape you have is not a flat disk but a cylinder. Estimates for the drag coefficient of a cylinder oriented so that the blunt end is perpendicular to the flow can be found in the classic book Fluid Dynamic Drag by Dr. Sighard Hoerner. According to the following graph, the coefficient of drag for a cylinder in this orientation is about 0.81 so long as the l/d (length-to-diameter ratio or fineness ratio) is greater than 2. As the fineness ratio shrinks to zero, the cylinder collapses to a flat circular disk. It is therefore not surprising that the drag coefficient for a cylinder with l/d=0 is about 1.17, the same as a flat disk. The reference area for a cylinder is also the same as that used for the disk except the radius is that of the cylinder's circular cross-section.

Drag coefficient of blunt nose and rounded nose cylinders versus fineness ratio l/d

The drag coefficient for a cone pointed into the airflow is a bit more complex since it depends on the cone's shape. In particular, the drag will vary depending on how steep the angle of the cone is. The angle of interest is called the half-vertex angle, ε, measured from the centerline of the cone to one of its walls. The larger this angle becomes, the higher the drag of the cone is.

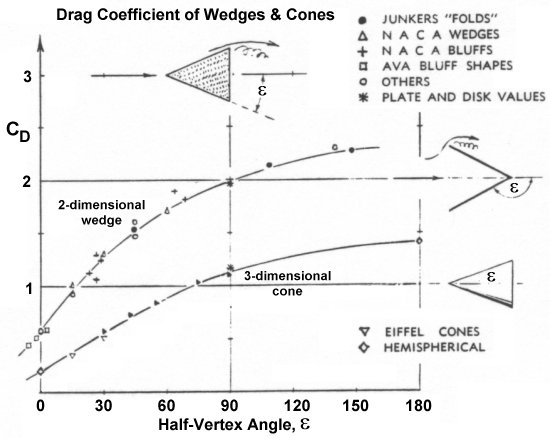
Drag coefficient of wedges and cones versus half-vertex angle

Based on the above graph provided by Hoerner, it appears that the drag coefficient is nearly linear with the half-vertex angle from 0° up to 90°. We can use this information to derive the following equation that closely approximates the experimental data for drag coefficient versus the half-vertex angle of the cone. Simply use the appropriate angle (in degrees) for the cone in your wind tunnel experiment to calculate the drag coefficient.

A half-vertex angle of 90° causes the cone to turn into a flat circular disk. As we have already seen, the drag coefficient of this shape is about 1.17. Plugging 90° into the above equation results in a drag coefficient of 1.17, exactly what we should expect at this particular angle. Again, the reference area for a cone is equal to the cross-sectional area of its base and can be calculated as π r².

Above 90°, the cone folds backwards and becomes hollow like a cup. The drag of this cupped shape remains fairly constant as the half-vertex angle of this cone increases to 180°. Typical drag coefficients of these and other basic shapes at Reynolds numbers between 10,000 and 1 million are compared in the following diagram.

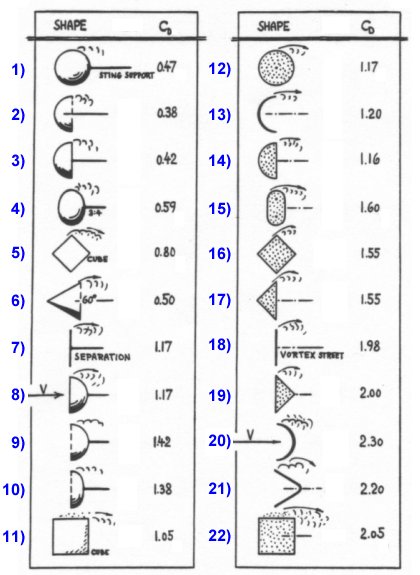
Drag coefficients of several simple 3D and 2D shapes

The table on the left compares three-dimensional shapes like disks, cones, and spheres while the table on the right is for two-dimensional shapes like plates, wedges, and cylinders. On the 3D side, note the flat circular disk in shape #7. The drag coefficient for this shape is given as 1.17, as we have discussed. The shape just above this one is a 60° cone, or a cone with a half-vertex angle of 30°. The drag coefficient of this shape is listed as 0.5. The simple equation we derived earlier predicts 0.498, a very close approximation.

Also note the two-dimensional cylinder in shape #12. This cylinder is oriented with its axis perpendicular to the flow rather than into the flow as described earlier. If a cylinder is mounted in this orientation in a wind tunnel, the drag coefficient should be about 1.17 using the same circular reference area assumed for all the shapes we have discussed.

This explanation has probably gone into much greater detail than was required, but understanding the drag characteristics of these simple shapes can often be very useful in predicting how more complicated objects behave. We have also explained the similarities between these different shapes, such as how they all collapse into flat disks and produce the same drag. Simple rules of thumb like these are often very useful as a method of quickly evaluating the accuracy of experimental data compared to theoretical predictions. Judgment skills of this kind can prove indispensable in the day-to-day work of an engineer.
- answer by Jeff Scott, 5 June 2005

Aircraft | Design | Ask Us | Shop | Search